Understanding Fractal Mathematics: A Journey Into Infinite Patterns

The Beauty of Fractals: An Introduction

Fractal mathematics is a fascinating field that captivates both mathematicians and artists alike. At its core, fractal mathematics is the study of shapes and patterns that repeat at different scales, creating intricate and infinitely complex structures. These patterns are not only visually stunning but also hold significant mathematical importance. Fractals can be found in nature, from the branching of trees and river networks to the structure of snowflakes and clouds. This natural occurrence makes fractals a bridge between mathematical theory and the real world, offering insights into complex systems that are otherwise challenging to understand. In this article, we will explore the basics of fractal mathematics, its applications, and why it continues to be a subject of intrigue and study in 2024.

What Are Fractals?

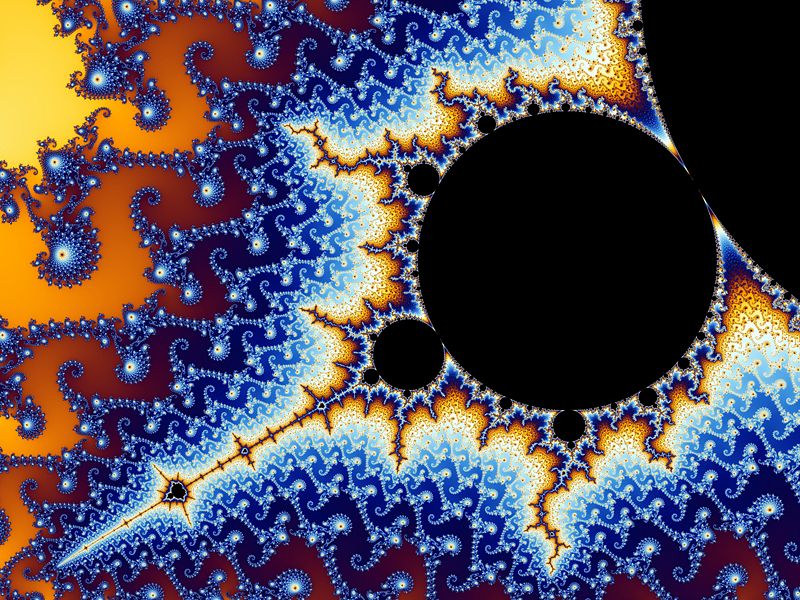
Fractals are geometric shapes that can be split into parts, each of which is a reduced-scale copy of the whole. This property is known as self-similarity. A classic example of a fractal is the Mandelbrot set, a set of complex numbers that forms a boundary exhibiting an infinitely complex, self-similar, and detailed pattern. The beauty of fractals lies in their ability to represent infinitely complex shapes with simple iterative processes. Despite their complexity, fractals are generated through simple, repetitive actions. This makes them a powerful tool for modeling and understanding phenomena that exhibit complexity and chaos. The concept of fractals extends beyond mathematics into fields such as computer graphics, art, and natural science, providing a universal language for describing and analyzing patterns.

The Mathematical Foundation of Fractals

The mathematical foundation of fractals is rooted in iterative processes and recursive algorithms. One of the earliest documented studies of fractals was conducted by Benoît Mandelbrot in the late 20th century, who coined the term "fractal" from the Latin word "fractus," meaning broken or fractured. Mandelbrot's work demonstrated that fractals could be used to model seemingly irregular yet intricate shapes found in nature. The mathematics behind fractals often involves complex numbers and functions, with iterations performed repeatedly to reveal the fractal structure. The concept of dimension is also crucial in fractal mathematics. Unlike traditional Euclidean geometry, where dimensions are whole numbers, fractals often have non-integer, or fractional, dimensions. This fractional dimension is a measure of the complexity of a fractal, providing a numerical value to describe its intricate patterns.

Exploring Famous Fractals

Several famous fractals have captivated mathematicians and artists alike. The Mandelbrot set, perhaps the most well-known fractal, is defined by a simple formula yet produces an infinitely complex boundary. The Julia set, related to the Mandelbrot set, is another example that exhibits stunningly complex shapes. The Sierpinski triangle and the Koch snowflake are classic examples of fractals constructed through geometric iteration. The Sierpinski triangle is formed by repeatedly removing equilateral triangles from a larger one, creating a pattern of nested triangles. The Koch snowflake, created by adding smaller triangles to each side of an initial triangle, results in a shape with an infinite perimeter but finite area. These fractals not only demonstrate the beauty of mathematical patterns but also serve as tools for exploring the concept of infinity and complexity in mathematics.

Applications of Fractals in Modern Technology

Fractals have found numerous applications in modern technology, particularly in fields requiring the modeling of complex and irregular structures. In computer graphics, fractals are used to create realistic landscapes, clouds, and textures through fractal-based algorithms. These algorithms allow for the efficient production of detailed images with minimal computational resources. In telecommunications, fractal-shaped antennas have been developed to improve signal reception and transmission. The self-similar structure of fractals enables antennas to operate efficiently at multiple frequencies, enhancing their performance. Fractals also play a role in data compression, where their mathematical properties are used to compress and decompress digital information. This is particularly useful in the storage and transmission of large data sets, such as images and videos. The versatility of fractals in technology underscores their significance beyond pure mathematics, driving innovation across various industries.

The Role of Fractals in Nature

Fractals are not only a mathematical curiosity but also a fundamental aspect of the natural world. Many natural phenomena exhibit fractal patterns, from the branching of trees and blood vessels to the formation of coastlines and mountain ranges. These natural fractals often arise from processes that involve growth, erosion, and diffusion, reflecting the underlying complexity and chaos of the natural environment. The study of fractals in nature provides valuable insights into ecological systems, weather patterns, and geological formations. For instance, understanding the fractal nature of river networks can aid in predicting flood patterns and managing water resources. Similarly, the fractal patterns found in plants and animals can inform studies on evolution, adaptation, and biodiversity. By examining the fractal structures in nature, scientists can gain a deeper understanding of the processes that shape our world.

Fractals in Art and Design

The aesthetic appeal of fractals has made them a popular subject in art and design. Artists and designers have long been inspired by the intricate and mesmerizing patterns of fractals, incorporating them into various forms of visual art. Fractal art is created using algorithms that generate fractal patterns, resulting in stunning images that showcase the beauty of mathematics. These artworks often explore themes of infinity, symmetry, and chaos, inviting viewers to appreciate the complexity and order inherent in fractal structures. In design, fractals are used to create visually engaging patterns for textiles, architecture, and digital media. Their self-similar nature allows for the creation of designs that are both harmonious and dynamic, appealing to a wide range of audiences. The intersection of fractals and art highlights the creative potential of mathematics, bridging the gap between science and aesthetics.

Challenges and Opportunities in Fractal Research

Despite the significant advances in fractal mathematics, there remain challenges and opportunities for further research. One of the primary challenges is the computational complexity involved in generating and analyzing fractals. The iterative processes required to create fractal patterns can be computationally intensive, necessitating advanced algorithms and computing power. Additionally, the study of fractals often involves complex mathematical concepts, such as chaos theory and non-linear dynamics, which can be difficult to grasp. However, these challenges also present opportunities for innovation and discovery. Advances in computing technology and mathematical modeling continue to expand the possibilities of fractal research, enabling the exploration of new applications and theoretical insights. The growing interest in fractals across disciplines, from physics and biology to art and design, suggests a promising future for this field of study.

The Future of Fractal Mathematics

As we look to the future, fractal mathematics is poised to play an increasingly important role in addressing complex problems and advancing technological innovation. The ability of fractals to model intricate patterns and systems makes them a valuable tool for understanding and predicting complex phenomena. In the coming years, we can expect to see further developments in the application of fractals to fields such as climate science, medicine, and artificial intelligence. For instance, fractals could be used to improve climate models by providing more accurate representations of atmospheric and oceanic patterns. In medicine, fractal analysis could enhance our understanding of biological processes and disease progression. The intersection of fractals and artificial intelligence also holds potential, with fractal algorithms contributing to the development of more efficient and adaptable AI systems. The future of fractal mathematics is bright, offering a wealth of opportunities for exploration and discovery.

Conclusion: Embracing the Complexity of Fractals

In conclusion, fractal mathematics is a field that continues to captivate and inspire, offering a unique perspective on the complexity of the world around us. From the stunning visual patterns they create to their practical applications in technology and science, fractals serve as a testament to the beauty and power of mathematics. As we move forward into 2024 and beyond, the study of fractals will undoubtedly continue to evolve, driven by advances in computing, mathematical theory, and interdisciplinary collaboration. By embracing the complexity of fractals, we can unlock new insights into natural and artificial systems, paving the way for innovation and discovery. Whether you are a mathematician, artist, or simply a curious mind, the world of fractals invites you to explore the infinite possibilities that lie within its intricate patterns.