Understanding Partial Derivatives: A Comprehensive Guide For 2024

Introduction to Partial Derivatives

Partial derivatives are a fundamental concept in the realm of calculus, particularly when dealing with functions of multiple variables. Unlike ordinary derivatives, which focus on functions of a single variable, partial derivatives allow us to explore the rate at which a function changes with respect to one variable while keeping others constant. This concept is crucial in fields such as physics, engineering, and economics, where systems often depend on multiple factors. In this guide, we'll delve into the nuances of partial derivatives, providing insights and tips to help you master this essential mathematical tool.

The Basics of Partial Derivatives

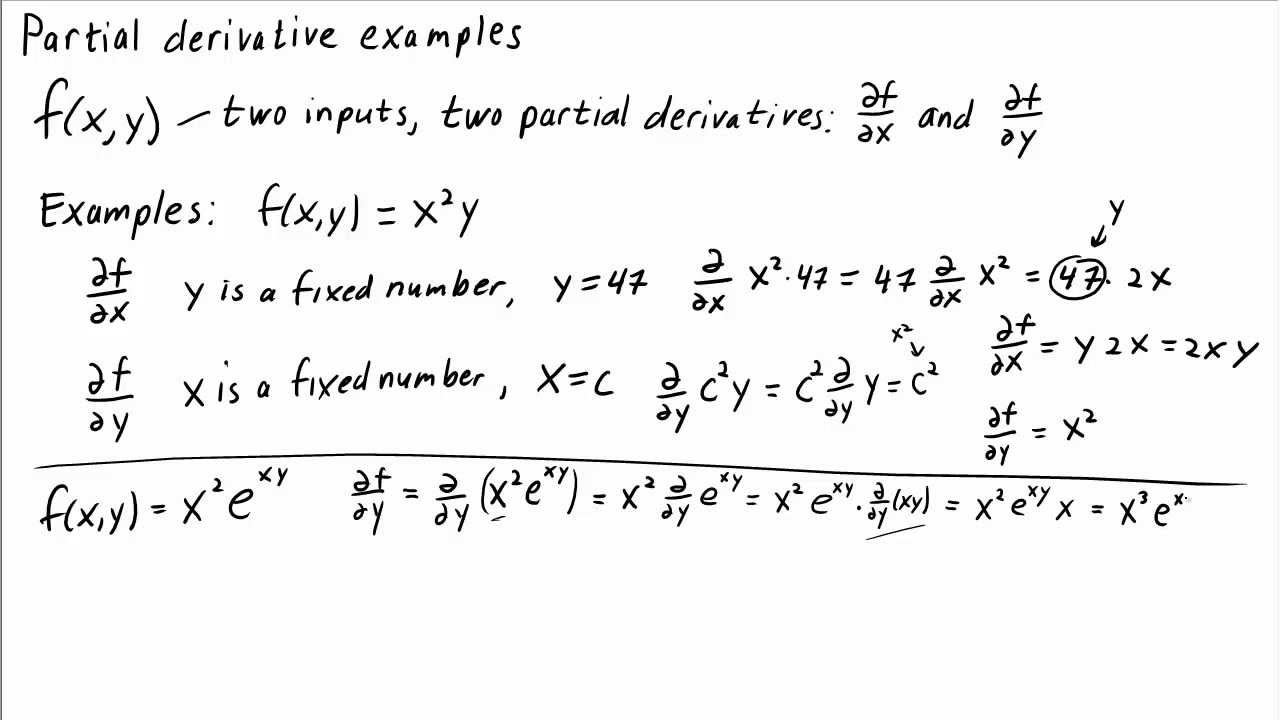
To grasp partial derivatives, it's essential to understand the concept of multivariable functions. Consider a function f(x, y) that depends on two variables, x and y. The partial derivative of f with respect to x, denoted as ∂f/∂x, measures how f changes as x changes while keeping y constant. Similarly, the partial derivative with respect to y, ∂f/∂y, examines the change in f as y varies, with x held constant. This approach allows us to isolate the effect of each variable on the overall function, providing a detailed view of its behavior.

Calculating Partial Derivatives

Calculating partial derivatives involves taking the derivative of a multivariable function with respect to one variable at a time. The process is similar to finding ordinary derivatives, but with extra attention to keeping other variables constant. For example, to find ∂f/∂x for a function f(x, y) = x^2y + y^3, treat y as a constant and differentiate the expression with respect to x. This yields 2xy. For ∂f/∂y, treat x as constant and differentiate with respect to y, resulting in x^2 + 3y^2. Practicing this with various functions will help solidify your understanding.

Applications of Partial Derivatives

Partial derivatives have a wide range of applications across different fields. In physics, they're used to describe how physical quantities change in space and time, such as temperature distribution in a solid object or the flow of fluids. In economics, partial derivatives help analyze how consumer demand changes with price while keeping other factors constant. Additionally, they're crucial in optimization problems, where the goal is to find maximum or minimum values of functions subject to constraints. Understanding these applications can provide valuable context and motivation for mastering partial derivatives.

Tips for Learning Partial Derivatives

Learning partial derivatives can be challenging, but with the right approach, it becomes manageable. First, ensure a solid understanding of ordinary derivatives, as this forms the foundation for partial derivatives. Practice with simple functions before tackling more complex ones, and use visualization tools to gain insights into how functions behave in multiple dimensions. Don't hesitate to seek help from textbooks, online resources, or tutors if you encounter difficulties. Consistent practice and application of concepts will ultimately lead to mastery.

Common Mistakes to Avoid

When learning partial derivatives, it's easy to make mistakes, especially for beginners. One common error is forgetting to treat other variables as constants when differentiating with respect to a specific variable. This oversight can lead to incorrect results and confusion. Additionally, when dealing with higher-order partial derivatives, ensure proper notation and differentiation order, as these can significantly affect the outcome. Recognizing and avoiding these pitfalls will streamline your learning process.

Higher-Order Partial Derivatives

Beyond first-order partial derivatives, higher-order partial derivatives provide deeper insights into the behavior of multivariable functions. These involve taking the derivative of a partial derivative. For instance, the second-order partial derivative ∂²f/∂x² measures how the rate of change of f with respect to x itself changes. Mixed partial derivatives, like ∂²f/∂x∂y, analyze how the rate of change with respect to one variable affects the rate of change with another. Understanding these derivatives is crucial for advanced applications and analysis.

Partial Derivative Notation and Symbols

In the realm of partial derivatives, notation plays a critical role in conveying information succinctly and accurately. The symbol ∂ is used to denote partial derivatives, distinguishing them from ordinary derivatives, which use the d symbol. When dealing with functions of more than two variables, subscript notation becomes essential. For example, ∂f/∂x is often written as f_x, and ∂²f/∂x² as f_xx. Familiarizing yourself with these symbols and conventions will enhance your ability to communicate mathematical ideas clearly.

Real-World Examples of Partial Derivatives

To appreciate the utility of partial derivatives, consider real-world scenarios where they play a pivotal role. In meteorology, partial derivatives help model atmospheric conditions by analyzing how temperature, pressure, and humidity interact. In finance, they assist in assessing the sensitivity of investment portfolios to changes in market variables. By understanding these applications, you can connect theoretical knowledge with practical uses, enriching your learning experience and highlighting the relevance of partial derivatives in everyday life.

Conclusion: Mastering Partial Derivatives

Mastering partial derivatives is a rewarding endeavor that opens doors to understanding complex systems and solving intricate problems. By building a strong foundation, practicing diligently, and applying concepts to real-world situations, you can develop a deep understanding of this essential mathematical tool. Remember to approach learning with patience and curiosity, embracing challenges as opportunities for growth. With dedication and perseverance, you'll find that partial derivatives become an invaluable asset in your mathematical toolkit, ready to be deployed whenever needed.